In preparation for Infinity Day which is on August 8th (or 8/8), I bring to you – 8 Cool Facts About Infinity! While I have always marveled at the wonders of this mathematical (and philosophical and cosmological and astronomical) paradox, it also first tends to bring to mind (the last decade or more) Buzz Lightyear! To infinity and beyond…. Did Shakespeare kind of, sort of know of this when he said ‘forever and a day?’ I wonder!

And keeping in tradition (my own), I also want to share books to do with infinity later in this post.

8 Cool Facts About Infinity

Well, potentially there are infinite cool things about infinity; but to start off, here are eight.

The Facts Themselves

There are as many even numbers as there are numbers; or to put it differently,

there are as many numbers that start with 581 or end with 296 as there are numbers; or there are as many odd numbers as there …. Well, you get the gist, right? For I can go on and on (infinitely, actually) to show this.

Conversely, some infinities are bigger than others (or smaller)

While, yes, all these varied sets are infinite, it kind of makes sense that the infinite set of only positive numbers is smaller than the infinite series of both positive and negative numbers. Or the infinite number of irrationals is bound to be larger than the infinite number of whole numbers. And of course, the infinite series of all numbers is bigger than those series that only include odd or even numbers, right? Right??

Infinity Plus One Is Infinity, or Hilbert’s Hotel Paradox

And then there is the way infinity allows for solving Hilbert’s hotel paradox which goes like this (in case you have not heard of it before):

Consider a hypothetical hotel with a “countably” infinite number of rooms, all of which are occupied. Now one new guest arrives. So therein lies the paradox. Can the hotel accomodate this new guest? In a normal hotel with a finite number of rooms, the answer would be no. But this hotel does have an infinite number of rooms, right? One might be tempted to think that the hotel would not be able to accommodate any newly arriving guests, as would be the case with a finite number of rooms.

The solution to this paradox: move guests up one room and have the new guest occupy room one.

What if more than one new guest shows up? There are answers to those as well. Suppose if ‘x’ number of guests arrive, have current guests move up by n (their current room number) + x rooms, so the first x rooms can be occupied by the new ‘x’ guests.

And what if an infinite number of new guests land at Hilbert’s? Look at this image below that shows what can be done then.

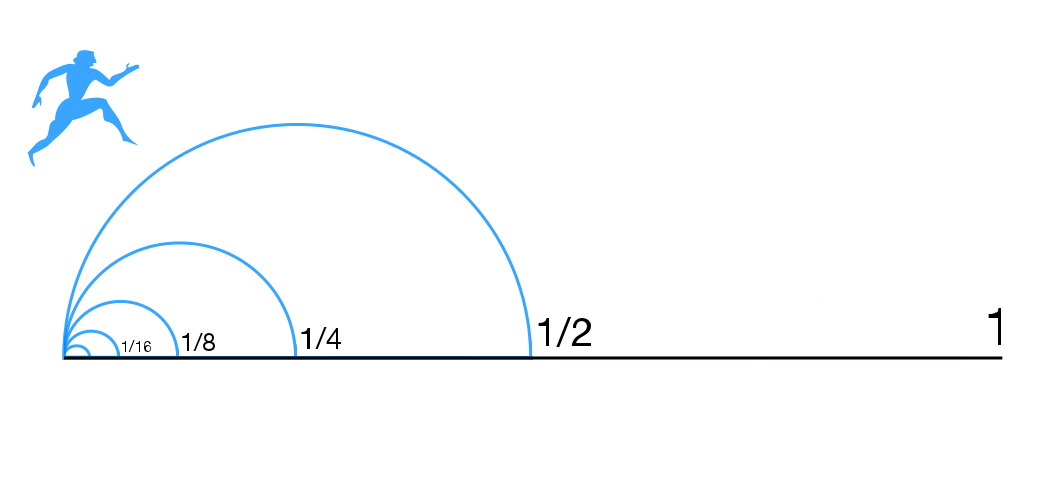
Zeno’s Paradox

While a distance or time-limit might be finite, it has an infinite number within. Say, you have to get to the table across the room which is x meters and about one minute away to get that piece of cake. Piece of cake, right? Just hold on. Before you can travel x meters, you have to first travel x/2 meters. And prior to that, you have to cover x/4, and so on, and on…

Similarly, before you walk for that one minute to reach the table, you have to walk for 1/2 a minute, and before that, for a quarter of a minute, and etc.

Both these sequences go on forever. Therefore, or in mathematical parlance, QED, you cannot cover the distance and there is always some little time left before you can accomplish your piece-of-cake goal.

This is Zeno’s Dichotomy Paradox (and even his Achilles and the Tortoise Paradox applies similar concepts)

Infinity Minus Infinity Does Not Equal Zero

What is 2-2? You know the answer: it is 0 (zero), of course! What about 12930334 – 12930334? Also zero!! But that does not apply to infinity. By now you should have guessed that infinity follows its own rules..

Let us check how..

We now have established (kind of) that:

infinity + 1 = infinity

Now, let us subtract both sides by infinity.

[infinity + 1] – infinity = [infinity] – infinity

If we use normal math rules, then the answer will end up as

1 = 0 [oops!!]

So it follows that (or hence proven) that

infinity – infinity ≠ zero

And then there is the Divide By Zero

If you check on your calculator (old-school or even on your mobile device), dividing by zero ends in ERROR; and when I tried it on an online calculator, it told me that I simply ‘Cannot Divide by Zero.’ But when we get into extended complex number theory, it shows that any number divided by zero is in fact, infinity. I recall learning this in high school myself, and thinking ‘WOW’!

And for reasons similar to why infinity minus infinity is not zero, or something divided by zero is infinity, infinity divided by infinity is not equal to one….. I need to read up more, I know that now!

What Does an Infinite Series Add Up to?

Now that we have tried subtraction and division, let us check addition as well. We all know that 1 + 1 is 2. Or even can work out something more complex (maybe using a calculator) like 98373 + 2985.

But do you know the sum of all positive integers 1+2+3+4…to infinity?? Well, Srinivasan Ramanujan did. And the answer is bound to surprise you.

Are you sitting down? Yes? Then, let me go ahead and tell you that the answer is -1/12, or rather,  -0.08333333333!!!!

You can read in the linked article in references section below…

The Infinite Monkey Theorem

I had to include this one here. Simply because…

The Infinite Monkey Theorem is a proposition that an unlimited number of monkeys, given typewriters and sufficient time, will eventually produce a particular text, such as Hamlet or even the complete works of Shakespeare. Or conversely, that given an infinite amount of time, one monkey, hitting keys at random on a typewriter keyboard will eventually produce that pre-mentioned text, be it the dictionary, or the Bard’s complete works.

And then the list can go on..

There are also fractals, the wondrous pi, the infinite cosmos, limitless stars in the universe, etc. But that is an infinite set of facts about infinity which would require an infinitely large post, so…..

A Quick Look at the History of Infinity Day

Infinity Day was conceived and created by Jean-Pierre Ady Fenyo, a sidewalk philosopher who came to be known as “The Original New York City Free Advice Man” (see The New Yorker magazine’s August 17, 1987 issue.)

And if you are wondering about the infinity symbol, check out this Wikipedia article.

References, Further Reading

For more facts about infinity, or simply to learn more, check out these sources below

• Wikipedia – Hilbert’s Hotel Paradox
• Working with Infinity: A Mathematical Perspective (PBS: NOVA)
• The Science and Philosophy of the Infinite (Dartmouth)
• Mathematicians Measure Infinities and Find They’re Equal
• Defending Zeno’s Paradox
• The Infinite Monkey Theorem
• The Ramanujan Summation: 1 + 2 + 3 + ⋯ + ∞ = -1/12?

Pin me

Infinity Reading List: The Books

This post contains Amazon and other affiliate links . If you purchase through an affiliate link, I may get a commission at no extra cost to you. Please see the full disclosure for more information. Thank you for supporting my blog.

The Boy Who Dreamed of Infinity: A Tale of the Genius Ramanujan

The Joy of x: A Guided Tour of Math, from One to Infinity 

And Now, the End of This Post

Dear reader, I would love to hear your thoughts on this post; and any other facts about infinity or numbers in general.